Given an array of integers heights representing the histogram's bar height where the width of each bar is 1, return the area of the largest rectangle in the histogram.
Example 1:
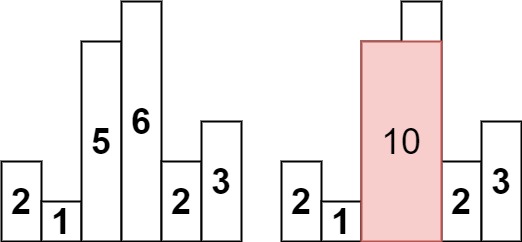
Input: heights = [2,1,5,6,2,3] Output: 10 Explanation: The above is a histogram where width of each bar is 1. The largest rectangle is shown in the red area, which has an area = 10 units.
Example 2:
Input: heights = [2,4] Output: 4
Constraints:
1 <= heights.length <= 1050 <= heights[i] <= 104
Companies:
Amazon, Microsoft, Adobe, Google, Facebook, Uber, Snapchat, HBO, ByteDance
Related Topics:
Array, Stack, Monotonic Stack
Similar Questions:
For a specific bar A[i], to form the largest rectangle we can form using A[i], we need to find the previous and next smaller element.
Assume their indexes are prevSmaller[i] and nextSmaller[i] respectively. So, A[prevSmaller[i]] is the index of the closest element to left of A[i] that is smaller than A[i], and A[nextSmaller[i]] is the index of the closest element to the right of A[i] that is smaller than A[i].
We can use similar solution as in 496. Next Greater Element I (Easy), i.e. Monotoic Stack, to get these prevSmaller and nextSmaller arrays.
Then for each A[i], the area of the max rectangle we can form using A[i] is (nextSmaller[i] - prevSmaller[i] - 1) * A[i].
In this implementation, we can precompute nextSmaller array and compute prevSmaller values on the fly.
// OJ: https://leetcode.com/problems/largest-rectangle-in-histogram/
// Author: github.com/lzl124631x
// Time: O(N)
// Space: O(N)
class Solution {
public:
int largestRectangleArea(vector<int>& A) {
int N = A.size(), ans = 0;
vector<int> nextSmaller(N);
stack<int> s; // strictly-increasing mono-stack
for (int i = N - 1; i >= 0; --i) {
while (s.size() && A[i] <= A[s.top()]) s.pop();
nextSmaller[i] = s.size() ? s.top() : N; // log nextSmaller for the current `i` on push
s.push(i);
}
s = {};
for (int i = 0; i < N; ++i) {
while (s.size() && A[i] <= A[s.top()]) s.pop();
int prevSmaller = s.size() ? s.top() : -1;
ans = max(ans, (nextSmaller[i] - prevSmaller - 1) * A[i]);
s.push(i);
}
return ans;
}
};Another variant:
// OJ: https://leetcode.com/problems/largest-rectangle-in-histogram/
// Author: github.com/lzl124631x
// Time: O(N)
// Space: O(N)
class Solution {
public:
int largestRectangleArea(vector<int>& A) {
int N = A.size(), ans = 0;
vector<int> prev(N, -1), next(N, N);
stack<int> s; // non-decreasing mono-stack
for (int i = 0; i < N; ++i) {
while (s.size() && A[i] < A[s.top()]) {
next[s.top()] = i; // log `next` of elements in stack on pop
s.pop();
}
s.push(i);
}
s = {};
for (int i = N - 1; i >= 0; --i) {
while (s.size() && A[i] < A[s.top()]) {
prev[s.top()] = i;
s.pop();
}
s.push(i);
}
for (int i = 0; i < N; ++i) {
ans = max(ans, A[i] * (next[i] - prev[i] - 1));
}
return ans;
}
};If we use the current A[i] as the right edge, when A[i] <= A[s.top()]:
- We use
A[s.top()]as the height of the rectangle s.pop()- The left edge
left = s.size() ? s.top() : - 1. - The area of this rectangle is
(i - left - 1) * height
// OJ: https://leetcode.com/problems/largest-rectangle-in-histogram/
// Author: github.com/lzl124631x
// Time: O(N)
// Space: O(N)
class Solution {
public:
int largestRectangleArea(vector<int>& A) {
A.push_back(0); // append a zero at the end so that we can pop all elements from the stack and calculate the corresponding areas
int N = A.size(), ans = 0;
stack<int> s; // strictly-increasing mono-stack
for (int i = 0; i < N; ++i) {
while (s.size() && A[i] <= A[s.top()]) { // Take `A[i]` as the right edge
int height = A[s.top()]; // Take the popped element as the height
s.pop();
int left = s.size() ? s.top() : -1; // Take the element left on the stack as the left edge
ans = max(ans, (i - left - 1) * height);
}
s.push(i);
}
return ans;
}
};We can use DP to compute the prevSmaller and nextSmaller arrays.
This is an O(N) solution because each computed prevSmaller/nextSmaller value is used at most once when computing a new prevSmaller/nextSmaller value.
// OJ: https://leetcode.com/problems/largest-rectangle-in-histogram/
// Author: github.com/lzl124631x
// Time: O(N)
// Space: O(N)
// Ref: https://leetcode.com/problems/largest-rectangle-in-histogram/discuss/28902/5ms-O(n)-Java-solution-explained-(beats-96)
class Solution {
public:
int largestRectangleArea(vector<int>& A) {
int N = A.size(), ans = 0;
vector<int> prevSmaller(N, -1), nextSmaller(N, N);
for (int i = N - 2; i >= 0; --i) {
int j = i + 1;
while (j < N && A[j] >= A[i]) j = nextSmaller[j];
nextSmaller[i] = j;
}
for (int i = 1; i < N; ++i) {
int j = i - 1;
while (j >= 0 && A[j] >= A[i]) j = prevSmaller[j];
prevSmaller[i] = j;
}
for (int i = 0; i < N; ++i) ans = max(ans, (nextSmaller[i] - prevSmaller[i] - 1) * A[i]);
return ans;
}
};